Age-related loss of cellularity in silico
Introduction
Gradual reduction of the amount of cells constituting postmitotic tissues appears to be one of the most reliable mechanisms realizing the program of ageing. A signal starting the program of cellular death (PCD) in a part of cellular population can be an excess of a threshold level of PCD inductor production as a result of stochastic processes. Speed of ageing is regulated by the proportion of average PCD inductor production, variability of this rate and the size of a threshold level of the PCD induction. The developed model allows to study the progress of these processes and their modulation by antioxidants in silico.
Source:
V. A. Chistyakov, Y. V. Denisenko (2009) Age-related cellularity loss in silico, Russian Journal of Chemisty. (The Journal of Russian Chemical D. I. Mendeleev Society), 53, 105–110.
The simultaneous coexistence a number of contradicting theories of ageing reflects system crisis of modern gerontology. One of the approaches making the construction of the unified theory of ageing easier, can be formation of some basic methodological framework based on obvious premises the majority of biologists accept. Because of the complexity of this problem (that is determined by complexity of the described phenomena) it is possible to suggest to solve partially, considering successively separate sides of the phenomenon of ageing in hope that this activity will facilitate the future synthesis. This work represents the attempt of realization of such approach as applied to to the phenomenon of cellularity loss in postmitotic tissues. Reduction of the number of cells composing tissues and bodies, seems to be one of the simplest and therefore reliable mechanisms of progressing with the years reducing resiliency of a multicellular organism. Importance of this process for ageing mammals is well enough shown [1, 2, 3, 4]. It is also obvious, that ageing of imago of diptera, submitting to the same statistical regularities, as ageing of the majority of other animals, is caused mainly by postmititic tissues ageing [5, 6].
By now enough facts are accumulated for construction of model of age cellularity loss, allowing to take into account delay of this process by geroprotectors. The preconditions of its logic are as follows:
• Postmitotic cells are capable to generate the substances provoking programmed cellular death (PCD) [7], - for example, hydrogen peroxide. We shall consider certain substance X (we would not like to concretize its nature up to the description of work of the mechanism of cellularity loss in general).
• At the increase of X quantity up to a certain limit produced during a time interval which can be named "cycle", the mechanism of self-liquidation works.
• The average X quantity, produced in cells during a cycle is lower than Ťa threshold of self-liquidationť.
• As X quantities for various cells are mutually weakly dependent, their distribution will submit to the normal law (Gaussian law). As it will be visible from the further statement, presence of asymmetry and excess at distribution of X size does not change analyzed laws essentially. The main thing is that function of distribution X at convergence of argument to infinity asymptotically tends to zero. As such curve does not cross the X-axis, there can be arbitrary large deviationsfrom average X size.
• In a cellular population, at an average level of X production that is lower than the threshold, there will be cells producing the amount of X which will exceed Ťthe threshold of self-liquidationť.
Let's take Uα size (fig. 1.) as the threshold X size at the excess of which the mechanism of self-liquidation is started. Then 1 - α (α - the area of light zone) will be equal to the proportion of cells dying during a cycle (as Gaussian curve in figure is normalized, the whole area under it is equal to 1). Shifting size Uα and/or changing σ (the standard deviation from X sizes distribution X size, for the normalized distribution σ = 1), experimenters or evolving alive systems receive an opportunity to adjust speed of cellularity loss, and, hence, the speed of ageing. Even the superficial visual analysis (fig. 1) convinces that insignificant shifts of Uα can cause big changes 1 - α or, concretizing the biological sense of considered processes, it is possible to assume that weak repeating influences, constant introduction of small geroprotector dozes for example, are capable to cause significant delay of ageing.
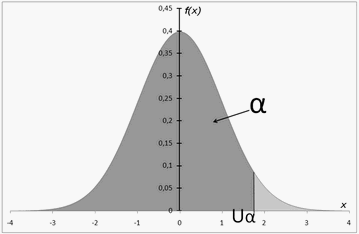
Fig. 1. The normalized curve of normal distribution.
Methods
For quantitative confirmation of the concept stated above the simulated multi-agent model with use of the approaches stated in work [8] was created. The model is realized in Java2 language. In this realization algorithms from the work [9] are used.
It should be noted that in trivial statement, when agents (cells and individuals) are passive and indistinguishable, the problem can be solved analytically, however application of the simulated multi-agent model allows to increase complexity of statement of the problem stepwise, without changing the model conceptually. Moreover, such approach does not assume aprioristic knowledge of laws of the system behaviour. On the contrary, the researcher can reveal global behaviour laws of a system (an organism) as a whole which are formed as result of interaction of agents with their environment and with each other, defining(determining) individual behaviour of the agent (a cell). Actually, revealing of such laws is also one of the purposes of the research.
It is possible to assume that multi-agent discrete decentralized stochastic and probably asynchronous model will reflect the behaviour of biological object more precisely than continuous analytical model [10].
In the presented work the multi-agent model consisting of 2000 agents was used, 1000 of agents participate in experiment, 1000 compose the control group. Time of existence of model in conditional cycles is set. Every agent is considered to produce some X substance, and its production during a cycle submits to the normal law of distribution with set standard deviation. Threshold value, the excess of which leads to destruction of the agent, is set. In the experiment, starting from some cycle, the mathematical expectation of production of X substance is reduced by some set size for the agent. Drawing biological analogies it is possible to say that the agent is given a preparation which reduces production of X substance according to schedule.
There is an opportunity of setting parameters for each agent (cell) individually, site of each agent, interaction between agents, etc., however for simplicity these opportunities at a first approximation were not used. It is possible to familiarize with work of this model here
Results and discussion
The typical screen layout after carrying out the computer modelling with use of the developed model is shown on fig. 2. The average size of X production in this case is 100 units, the standard deviation - 20 units, threshold value - 158 units, reduction of the average value because of introduction of the preparation - 5 units. A preparation is started to be given from the 200-th cycle. The bottom curve describes cellularity loss in control group, the top one - in experimental group.It can be seen from the figure that though threshold value exceeds average X production in 1,58 times or approximately in 3σ, about 2 cells from the control population die during each cycle.
 А
А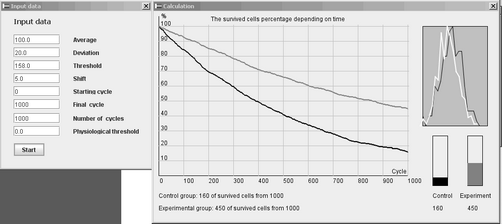 Б
Б
Fig. 2. A screen layout at carrying computer modelling out with using the developed model. A - introduction of the protector from the 200th cycle, B - from a zero cycle.
Up to 200-th cycle (the moment of introduction of the protector) the destruction of agents in control and experimental groups is approximately identical. By the 200-th cycle the survival rate in both groups worked out at about 68 % (32 % of agents were lost). From 200-th cycle when the preparation was applied, the survival rate in experimental group has appreciablly increased. By the 371-st cycle in control group half of agents was lost by that moment. In experimental group about 39 % of agents were lost. At introduction of the protector causing similar shift from the zero cycle, the difference in survival rate of the experimental and control populations works out at 24,5 % by the 367-th cycle. By the 800-th cycle half of the experimental group population survives.
It is remarkable, that such significant increase of survival rate is gained by the action of the protector lowering the average X value by 5 units (5 %). This shift practically is not distincted by means of variational statistics methods (see curves of distributions in the top right corner of the figure). Thus, the imitating approach allows to create models of functional cumulation process at which superweak multiply repeated influences, each beiong statistically indiscernible, lead to to significant physiological effect.
Even this imperfect version of the model developed by us seems to be suitable for modelling physiological effects of geroprotectors. Lets assume that the analyzed population is represented by cells of some gland of a mouse, and duration of a cycle is equal to one day. Supposing that 60 % cell loss conducts to production decrease of a secret which initiates mice vibrussae loss. In the conditions displayed on fig. 2, control animals wil start losing vibrissae from the 500-th day of life what is close to the real situation. Model experiment shows (tab. 1), what even 2 % X shift grants an appreciable delay of beginning of vibrissae loss. Five-percentage reduction of average intensity of PCD inductor production conducts to a delay of this process so that it starts somewhere out of limits of a life of Ťthe virtual mouseť - 1000 cycles. It should be noted that this situation reminds the action of SkQ1 [11, 12]. Mice ingesting it do not lose their vibrissae during all term of a life.
| Size of X shift, % | A delay of 60 % of cellularity loss, cycles |
| 2 | 200 |
| 3 | 240 |
| 4 | 400 |
| 5 | >500 |
There is a set of the examples convincin that if the increase in life expectancy of individuals promotes prosperity of species, alive systems can reach it quickly enough (on the evolutionary time scale) i.e. selection for the increase of life expectancy can be rather effective.
Shift of the threshold of self-liquidation towards greater values can be one of possible ways to delay the speed of cellularity loss of postmitotic tissues used by living organisms. Cells of such rodents - long-livers, as deer-mouse and naked mole rat are known to possess a greater stability to hydrogen peroxide compared to Mus musculus [13, 11].
Administring antioxidants we shift X towards reduction. Here the same law works. As value of programmed death threshold is set genetically and is hardly shifted by antioxidant administration, rather a small X reduction will be also accompanied by significant speed reduction of cell dying and, hence, ageing speed reduction as in the previous case. Therefore the action of the antioxidant which is aimed to slow ageing down, should be not powerful but continual, so that the effect would not be lost during long administration. Modelling shows that short-term administration of the protectors completely overwhelming cellularity loss during 1 - 100 cycles practically does not change general dynamics of the process. Apparently the prolonged effect of SkQ1 created during the Russian gerontological megaproject [11] is its main advantage over other antioxidants - geroprotectors.
Researches of the SkQ effect on a fruit fly generally confirmed actuality of of models predictions. Thus the preparation administration during a week in the middle of life was inefficient, while its prolonged reception during the second half of life prolonged its term. However a phenomenon which has not been succesefully simulated yet was discovered as follows: geropritective effects similarity in short-term SkQ1 introduction in the first week and constant introduction of the preparation [12]. Probably, we will manage to liquidate this disadvantage by supplying virtual cellsa certain genetic device capable of being damaged.
Nevertheless, the approach used by us allows to illustrate importance of optimization of preparation administration pattern to achieve the peak efficiency. The modelling results of antioxidant administration during zero to the 500-th cycles (A) and from the 500=th to the 1000-th cycle are represented on fig. 3. As the figure shows, administration of same total protector quantity during the different periods of life practically does not change the final results of survival rate in the experimental and in the control groups. So the increase of life expectancy of a virtual population of cells practically does not depend on the order of preparation adminisration. However the area under the curve essentially depends on this parameter, reflecting the general number of celular cycles during the researched period. This parameter can have a number of biological parallels. In particular if we assume that the thymocyte population is modelled the area under the curve will correspond to the integrated parameter of a level of potential immune answer force, etc.. In general the area under the curve correlates with certain total viability, determining life quality. Thus the power of long acting geroprotector physiological effects can be determined not only by only its quantity, but also by the pattern of preparation administration.
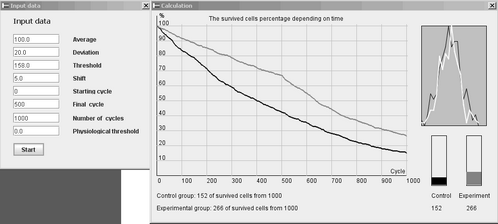 А
А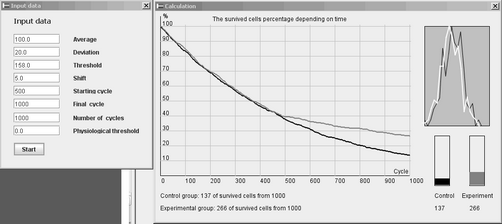 Б
Б
Fig. 3. Modelling of geroprotector adminisration from zero to the 500th (Ŕ) and from the 500th to the 1000th (B) cycles.
In the theoretical constructions the modern biochemistry operates basically by average magnitudes. Parameters of a variation are used, as a rule, for the estimation of the statistical importance of average value distinctions. Meanwhile, the reduction of scatter of X determined by the standard deviation i.e. accuracy increase of cellular bio-energetics work can be another way to reduce the speed of cellularity loss in postmititic tissues.
As the modelling experiments carried out with the parameters, represented on fig. 2 show, insignificant σ reduction leads to to noticeable delay of cellularity loss at constant average manufacture of X substance (tab. 2). In connection with this it is interesting, that life expectancy variation factor of mice receiving SkQ1 is approximately 30 % lower than control value (see tab. 1 in the article [12]. The point of hos these distinctions can reflect differencies in intercellular variations of ROSs generation, is certainly actual and demands separate research.
| Standard deviation | Moment of 50 % cell destruction achievement, cycles |
| 21 | 230 |
| 20 | 380 |
| 19 | 640 |
| 18 | 1000 |
Apparently, the program of multicellular organisms ageing is partially set by X, σ and Uα (a threshold of self-liquidation) balance in different tissues, basically, in gland tissues. These processes determine the self-destruction pattern of an organism which can vary as a result of genetically determined features of particular glands and tissues ageing. There are many examples of people living up to the old age without losing their mental alertness, teeth, hair or hair greying, etc. Nevertheless, the probability of the ageing program failure is extremely low. High complexity of living system extremely complicates simulation of real variety of modelled objects properties in silico. Therefore the value of such researches is to a large extent determined by their ability to form a heuristic basis for experimental biology, that is to produce ideas for new experiments and to originate nontrivial interpretations of the results of ones carried out before. In this respect it should be mentioned that geroprotector overdose can be a serious reason of their low efficiency. As mentioned above, the antioxidant effect constancy at chronic administration can be more important for the antioxidant-geroprotector efficiency than its "power". We believe that in the majority of gerontological experiments dozes not yet toxical, but already starting cellular mechanisms of correction antioxidant status towards reduction are used. V.P.Skulachev called researchers attention to the existence of such mechanisms repeatedly. The creation of substances such as SkQ, efficient in nanomolar concentrations became the successful attempt to elude them [11,14]. Efficiency SkQ is determined by its ability to accumulate in mitochondria. Proceeding from the above-stated logic even nanomolar dozes of SkQ tested by now, kilograms of animals weight equivalent, can be superfluous, just as milli-and micromolar dozes of such antioxidants - geroprotectors, as tocopherol, mercaptoethanol, etc. Progress of Ťantioxidant geriatricsť in many respects will be conditioned by creation of highly effective mixes. It is obvious that minimization of concentration of the working substances is one of the success preconditions in achievement of desirable efficiency.
In conclusion we would like to consider the correspondence problem of the distribution parameters used by us to real distributions of intercellular variations. As mentioned above, modern biochemistry, and for a wonder, cytology are concentrated basically on the analysis of averages. Nevertheless, there is a number of works in which intercellular variations are analyzed in detail. The frequency histogram of neutrophil distribution according to the level of generation superoxide anion during the respiratory explosion induced by phorbol dibutyrate is given in the article [15]. The data on the significance level more than 0,6 (The Pirson criterion) correspond to logarithmically normal distribution with characteristics μ = 2,25 and σ = 0,24.
Application results of visualization technique of the separate molecules arrangement of mRNA are published in the work [16]. As it can be seen from the given photos, even genetically identical cultural cells vary considerably in their contents. The authors believe that these distinctions smooth out at protein synthesis. Nevertheless, the frequency histogram of cell distribution according to the amount of a yellow fluorescing protein corresponds to normal distribution with characteristics μ = 0,31 and σ = 0,14. Thus, the in silico balance between the average and the standard deviation used in our experiments corresponds to the order of magnitudes existing in the nature.
The materials given in this article are the reflection of rough modelling of stochastic processes participation in of ageing control support. It is necessary to take into account inter- and intratissue heterogeneity of cellular populations, influence of cells upon each other. Besides PCD inductors in subthreshold concentrations can damage DNA, starting the processes fraught with death of cells. Nevertheless, the opportunities of imitating modelling are far from used up - its apparatus will allow taking into account the above-named characteristics in more complex models. Authors express profound gratitude to Vladimir Petrovich Skulachev and Vasily Nikolaevich Manskih for the ideas expressed when preparing and discussing the article.
Reference list
1. Anisimov V.N. Molecular and physiological mechanisms of aging. St. Petersburg: Nauka, 2003, 468 p.
2. Gruver A.L., Hudson L.L., Sempowski G.D. J. Pathol. 2007, V.211, š2, P. 144-156.
3. Jung T., Bader N., Grune T. Ann N Y Acad Sci. 2007, š1119, P.97-111.
4. Krishnan K.J., Reeve A.K., Samuels D.C., Chinnery P.F., Blackwood J.K., Taylor R.W., Wanrooij S., Spelbrink J.N., Lightowlers R.N., Turnbull D.M. Nat Genet. 2008, V.40, š.3, Đ.275-279.
5. Miquel J. Exp Gerontol. 1998, V.33, N1-2, P. 113-126.
6. Miquel J. Ann N Y Acad Sci. 2002, V. 959, P. 508-516.
7. Manskikh V. N. Tsitologiya 2007, V. 49, N. 11, P. 909-915.
8. Karpov Y.G. Imitating system modelling. Introduction to modelling with AnyLogic 5. BHV-St. Petersburg, 2005, P. 291-310.
9. D. E. Knuth. The Art of Computer Programming. Seminumerical Algorithms, Third Edition. Massachusetts: Addison-Wesley, 1997, 762p.
10. M. T. Jones. Aartificial Intellect Application Programming (Programming Series), 2003. Charles River Media. 363pp.
11. Skulachev V.P. Biochemistry (Mosc) 2007, V. 72, N12, P. 1572 - 1586.
12. V. N. Anisimov, L. E. Bakeeva, P. A. Egormin et al. Biochemistry (Mosc) 2008, V. 73, š 12, P. 1665 - 1670.
13. Csiszar, N. Labinskyy, X. Zhao, F. Hu, S. Serpillon, Z. Huang,P. Ballabh, R. J. Levy,T. H. Hintze, M. S. Wolin, S. N. Austad, A. Podlutsky , Z. Ungvari. Aging Cell 2007, V.6, š 6, P. 783 - 797.
14. Antonenko YN, Avetisyan AV, Bakeeva LE et al. Biochemistry (Mosc) 2008, V. 73, š 12, P. 1589 - 1606.
15. Szucs S., Vámosi G., Póka R., Sárváry A., Bárdos H., Balázs M., Kappelmayer J., Tóth L., Szöllosi J., Adány R. Cytometry 1998, V 33, N1, P. 19-31.
16. Raj A., Peskin C.S., Tranchina D., Vargas D.Y., Tyagi S. PLoS Biol. 2006, V. 4, š10, P. 309.