Simulation of Drosophila aging in silico
Introduction
One of the main tasks of modern gerontology is isolation of a group of phenomena essential for aging from epiphenomenona accompanying them. The modern methods of simulation modeling may be the proper tool for correct isolation. The point of their application is reproduction of external aging manifestations in silico, and, in the first place, of standard survival curves with the help of the simplest internal mechanism. It seems that such a mechanism will be prime mover of aging. The aging model of one the most popular objects of experimental biology - a fruit fly (Drosophila melanogaster) created by us , is based on the assumption that the key mechanism of aging is cellularity loss, the speed of which is determined by the parameters of active oxygen forms generation in motoneurons.
Source:
V. A. Chistyakov, Y. V. Denisenko (2010), Simulation of Drosophila aging in silico, Advances in Gerontology, 23, 557–563.
Aging is an amazing phenomenon and in the course of biology development scientists get more and more interested in studying it. The most intriguing question of this field of knowledge can be formulated as follows: what mechanism provides gradual decrease in viability of a multicellular organism? Development of experimental biology methods aroused a desire to answer it, studying the molecular changes which accompany the aging process.
Researchers have revealed many such changes starting from lipofuscin accumulation to telomere shortening [1]. Moreover, the system of mechanisms of the somatic cells programmed death was discovered and its participation in "self-destruction" of the whole organism was proved by the number of observations [5, 14]. The success in the search of processes characteristics of which correlate with age also had a reverse side. A lot of aging theories exaggerating the value of some or another molecular change have sprung up. Redundancy of the existing aging hypotheses pool is evident to the majority of authors trying to analyze the state of things in gerontology [1].
A multicellular organism is a multilevel self-organizing system. Activity of its elements is coordinated by the most complicated network of feedbacks. Any action is accompanied by a loop of gene expression changes, synthesis of hormones, immune and electrophysiological reactions, etc. The majority of them influences fitness. Nevertheless, for understanding of the processes taking place, for example, at digestion of food it is enough to keep in mind not more than ten physiological and biochemical reactions.
The dialectics of the cognitive process is such that abstraction from the majority of details allows creating conceptual models which become a basis of the further detailed elaboration, but already without any loss of understanding of the essence of the phenomenon studied. The theorist who is searching for the main laws of biological processes can achieve success only referring the majority of reactions accompanying them to epiphenomena.
Distinguishing the group of phenomena essential for aging from epiphenomena accompanying them is one of the main tasks of modern gerontology. We believe that modern methods of mathematical modeling can be the tool for distinguishing them properly. The sense of their application is reproduction of the "external" aging manifestations in silico, first of all of the standard survivor curves , by means of the simplest Ťinternal mechanismť. This mechanism will apparently be the most essential aging mover. Naturally "the internal mechanismť modeling should be based on the biological facts without contradicting them.
Creating the aging model of one of the most popular experimental biology objects, a fruit fly (Drosophila melanogaster) based on the simplest presuppositions possible was the task of this research.
Drosophila melanogaster life expectancy is measured by several weeks. This organism is an insect with complete metamorphosis. All somatic cells of an imago are postmitotic. Nevertheless, drosophila Ťsurvivor curvesť have the S-shape characteristic for the majority of animals [8, 12]. Aging modeling of the given object is essentially facilitated by the fact that cells responsible for this process are identified reliably enough and that the key role of the free-radical processes in aging [12] is clearly demonstrated.
As it is known, the basic source of reactive oxygen species in eukaryotic cells is a respiratory chain of mitochondria. One of the main enzymes-antioxidants is superoxide dismutase (SOD), catalyzing superoxide anion transformation into hydro peroxide and oxygen. It is known that the drosophilae, incapable of SOD synthesis are characterized by short life expectancy [16].
In the early nineties of the last century scientists succeeded in breeding drosophila, having appreciable quantities of human SOD, however, their life expectancy practically didn't differ from that of the wild type. Then the technique appeared that allowed to "switch on" SOD expression only in the necessary tissues by means of additional genetic construction. Specific expression of this gene in motoneurons turned out to make life expectancy longer. Moreover, human SOD introduction into motoneurons only returned life expectancy characteristic for the wild type to the flies defective in this enzyme.
Motoneurons are convenient for modeling because of the fact that their quantity in one fly is defined precisely enough and is about 80 [11]. It is possible to assume that the natural death of a drosophila occurs due to the motoneurons number decrease to a critical level.
The model of cellularity loss of a homogeneous population of postmitotic cells described in our work [7]
has served as the basis for creation of the drosophila aging model. Its logical bases are as follows:
Postmitotic cells are capable to generate a number of substances provoking programmed cellular death (PCD) - for example, hydrogen peroxide. Let us call X the total concentration of such apoptosis signals.
At the increase of X quantity up to a certain limit produced during a time interval which can be named a Ťcycleť( in this particular case the length of one cycle is 24 hours), the mechanism of self-liquidation works
The average X quantity, produced in cells during a cycle is lower than Ťa threshold of self-liquidationť.
As X quantities for various cells are weakly interdependent, they will be distributed according to the normal law (Gaussian law). The presence of an asymmetry and an excess at the X distribution does not change the analyzed laws essentially. The main thing is that the function of X distribution at convergence of argument to infinity asymptotically approaches zero. As this curve does not cross the X-axis, there can be arbitrary large deviations from average X size.
In a cellular population, at an average level of X production that is lower than the threshold, there will be cells producing the amount of X which will exceed Ťthe threshold of self-liquidationť.
The proportion of Ťself-liquidatingť cells during one cycle, and, hence the speed of cellularity loss, depends on the average size and a standard X deviation and level of Ťa self-destruction thresholdť
The modification of the model created for the drosophila aging modeling allows to take into account the death of flies reaching some critical level of motoneurons loss.
Materials and methods.
For the quantitative analysis of the described concept the multiagent imitating model of flies population has been created. The model is realized on Java 2 platform. In the realization of the model the algorithms from the work [3] were used.
During model creation organisms (flies) are formed as independent agents. In the simplest studied case such agents do not interact with the environment and with one another. Each fly bears the amount of motoneurons set by the experimenter.
The behavior of motoneurons submits to the following logic: it is considered that each motoneuron produces X substance, and its production during the cycle is regulated by the normal distribution law with the parameters that can be set. Threshold value of the Ő quantity is also set and exceeding it leads to motoneuron destruction.
When the agreed percentage of motoneurons destruction in each separate organism is achieved the fly dies. Thus, observing of the set of the agents formed according to the stated rules, it is possible to draw a survivor curve for flies population.
In the course of the experiment two populations of the flies are formed one of which is the control one and the second is exposed to the influence of the geroprotector, changing development of a substance X. The geroprotector introduction schedule is set by the experimenter. The size of control and experimental populations is limited by technical possibilities of a computing platform.
Program realization of the model is supplied by user-friendly interface and demands from the potential experimenter the minimum skills of work with computer technics. There is a possibility to vary the parameters mentioned above, or to make computational experiment with parameters by default.
It is possible to familiarize with work of the full-function version of this model here
Results.
Survivor curves of wild type flies and of flies with additional superoxide dismutase activity in motoneurons from this work [12] are presented on fig. 1A.
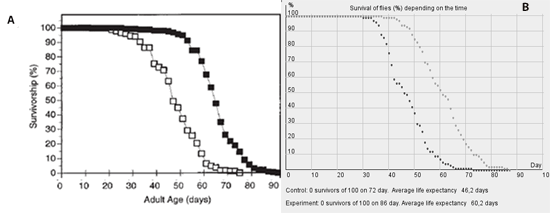
Fig. 1. A experimental survivor curves of wild type flies (empty squares) and of flies with additional SOD expression (black squares); B virtual survivor curves of wild type flies (black dots) and of flies with additional SOD expression (gray dots)
As the figures show, in spite of short life expectancy, experimental drosophilas survivor curves show elements, characteristic for the universal survivor curve for multicellular organisms, including the human Gompertz-Mejkham curve [10]. It includes the youth period (days 0-30) when the survival rate doesn't fall considerably with the years; maturity-old age period (days 30-60) when the survival rate starts to drop quickly; extreme old age period (days 60-80) when survival rate drops slower.
Fig. 1B shows the typical picture displayed on the screen after carrying out computer modeling with the use of the developed model. The size of the population is in this case 100 flies in the experimental and the control groups. One fly has 80 motoneurons. 40 % of them (32 motoneurons) are enough to survive. The average size of X (apoptosis inductors) production is 99 units, a deviation is 20 units and threshold value is 141 units, reduction of the average because of preparation application 2 units.
Comparison of figures 1A and 1B shows that with such parameters our model allows to reproduce real experimental dependences in silico almost identically.
It is interesting that for an increase of drosophila life expectancy reached with the help of additional SOD expression insignificant decrease (about 2 %) of level of apoptosis inductors production is required. It corresponds to the idea that SOD is a highly specialized tool of antioxidant protection, the efficiency of which is rather low without the complex system with catalase, glutathione-peroxidase and low-molecular antioxidants.
As it has already been mentioned before, our model simulates not only chronic, but also short-term geroprotectors introduction. It allows to cast light on the reasons of efficiency differences of antioxidants introduction during different periods of drosophila life. In the experiments with Skulachev ions with antioxidant charge it was shown that efficiency of these preparations is maximum if they are introduced during the first days of life, and then it falls dramatically [2]. Fig. 2 shows the relation between drosophila life expectancy increase and the moment of preparation introduction received for our model. It can be seen that the given relation is reflected by an s-symmetric curve, with a curve bend at the period of 30 50 days.

Fig. 2. Modeling of drosophila average life expectancy (LE) increase at introduction of the antioxidant reducing apoptosis inductors production in motoneurons by 2 %, in different decades of imago life, the confidence 5 % interval is given. Out of ten replications the average ones are shown.
The efficiency of preparation introduction in the "youth" period is much higher, than in the following periods. Its introduction in the first decade of life results in the same increase of average life expectancy, as its introduction during the days 35-100 (see fig. 3). Preparation introduction in the second half of life is almost inefficient. Comparison of the in vivo and in silico experiments allows to claim that the phenomena discovered in the work [2] are not connected to the age changes in preparation assimilation or in its efficiency at the molecular level, but are the consequence of statistical regularities.
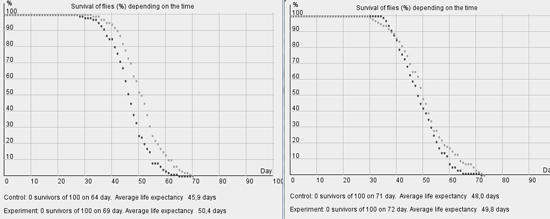
Fig. 3.Modeling of antioxidant introduction on the days from 1 to 10 (A) and from 35 to 100 (B) of drosophila life.
Discussion.
As we have already mentioned above, the experiments described in the work [12] have reliably shown the role of motoneurons and the importance of free-radical oxidation processes in drosophila aging. Roughly speaking, drosophila aging can be provided by: the reduction of motoneurons number, the decrease in their work efficiency, the combination of the first and second mechanisms. The results received by means of our model, have shown that for reproduction of normal flies real survivor curves and flies with additional SOD survivor curves, and also of experiments with introduction of antioxidants into drosophila forage, it is enough to consider only cellularity loss.
For the first time the defining role of postmitotic tissues cellularity loss in the aging mechanism was postulated by outstanding physicist Leo Scilard [15]. Using the mathematical apparatus he developed for the problems of nuclear physics, in particular for calculation of critical mass, Scilard has shown that cell destruction at the expense of casual internal events can provide age viability decrease of an organism with dynamics coinciding with that observed in actual practice. Scilard introduced a special concept -Ťŕging hitť for an event which leads to death of a cell.
As his work was written in 1959 when practically nothing was known about programmed cellular death, inactivation of genes necessary for cell survival was supposed to be the reason of the aging hit. It was a controversial point even then. In particular it is difficult to explain on the basis of genetic defects accumulation how the speed of aging necessary for a species survival is adjusted. There is a number of the examples convincing that when the increase of life expectancy of individuals promotes species well-being, living systems can reach it rather quickly (on evolutionary time scale), i.e. selection based on life expectancy increase can be rather effective. It is known, for example, that average life expectancy of individual and social rodents having equal weight differs dramatically.
The similar picture is characteristic for insects. Single wasps from whom ants descended, live no longer than one season, and the maximum registered life expectancy of a redwood ant queen is about 18 years. Average human life expectancy exceeds that of a chimpanzee (in mild zooconditions) more than twice, although they have 98 % of similar genomes. There are a lot of such examples. However, irrespective of the hypothetical mechanism, the idea of Ťaging hitť is a brilliant example of advanced quantum physics methodology introduction into biology.
The conception of aging hit as a result of cells with high values of natural fluctuations of apoptosis inductors production leaving into apoptosis offered by us, does not have the above-mentioned disadvantages, local genetic changes are enough for the "adjustment" of the necessary antioxidant activity level and the apoptosis threshold. Anyway, the domestic long-liver rodents close due to the evolution - the deer mouse and the naked mole rat are characterized by high cell resistance to hydrogen peroxide [6, 9]. Our model shows that only it already allows to explain their slower aging hypothetically.
The approach developed by us allows to make a number of conclusions necessary for explanation of an interesting gerontological phenomenon from life of the insects dramatic distinctions of life expectancy between working and fertile individuals of social animal species. Life expectancy of worker-bee is several weeks, and that of the queen-bee is up to 5 years. At the same time from the genetic point of view worker-bee sand the queen-bee from one hive are twins [13].
As the computing experiments show, the essential parameter for aging speed during the work of the mechanism simulated by us is the correlation of the threshold size of apoptosis start, the average value of apoptosis inductors production level and the standard deviation, and the influence of the threshold size is especially impressive. For example, an increase of the threshold size from 141 to 174,5 (less than by 24 %) in the experiment, the results of which are given in Fig. 2B (all else equal), leads to an increase of an average life expectancy of individuals up to 10000 days (see fig. 4). In the work [13] the facts testifying that queen-bees are considerably more resistant to lethal action of the intracellular superoxide anion generator paraquat than worker-bees and it can be connected both with the higher activity of antioxidant mechanisms, and with the increase of the threshold size of cell transition into apoptosis.
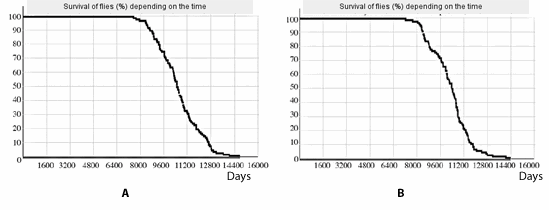
Fig. 4. Modeling of drosophila aging at an increase of the apoptosis threshold size by 24 % (A) or at a decrease of the standard deviation of apoptosis signals production by 44 % (B) Initial parameters of model are similar to those in fig. 1B.
This size depends on sensitivity and concentration of the cellular ROS detectors, the most well-known of which is đ53 protein. It is known, however, that loss of đ53 protein sensitivity to ROS is fraught with carcinogenesis induction [14]. In the course of the process of evolution this side effect can be overcome by the development of apoptosis independent mechanisms of protection against cancer. For medicine the way of aging retardation, caused by the loss of sensitivity to apoptosis signals, is, apparently, a deadlock.
Modeling shows that considerable decrease of aging speed can be achieved by a decrease of intercellular variations of apoptosis signals level, without changing their average level and the threshold size of leaving into apoptosis. What systems define the level of ROS production variation by separate cells is not known today. Probably, this factor is under biological (genetical) control as it is one of the key factors for interaction of cells in a multicellular organism. Studying this question is of great theoretical value, and for the problems of practical gerontology it is, apparently, one of the key questions.
This work is completed with the financial support of the Ministry of Education and Science of the Russian Federation (ŤDevelopment of scientific potential of the higher school (2009-2010) ťgrant š 2.1.1/5630)
References
1. Anisimov V.N., Russian Journal of General Chemistry, 2010, Vol. 80, No. 7, pp. 1019.
2. Anisimov, V.N., Bakeeva, L.E., Egormin, P.A., et al., Biochemistry (Moscow), 2008, vol. 73, no. 12, pp. 16651670.
3. Knuth, D.E., The Art of Computer Programming. Seminumerical Algorithms, Massachusetts: Addison-Wesley, 1997, 3rd ed.
4. Manskikh, V.N., Tsitologiya, 2007, vol. 49, no. 11, pp. 909915.
5. Severin F. F., Skulachev V. P., Advanced in gerontology 2009 vol. 22 no. 1 pp. 37 48.
6. Skulachev, V.P., Biochemistry (Moscow), 2007, vol. 72, no. 12, pp. 15721586.
7. Chistyakov V.A., Denisenko Yu. V., Russian Journal of General Chemistry, 2010, Vol. 80, No. 7, pp. 15011506.
8. Bahadorani S., Bahadorani P., Phillips J.P., Hilliker A.J. The effects of vitamin supplementation on Drosophila life span under normoxia and under oxidative stress.// J. Gerontol A Biol Sci Med Sci. 2008 Jan;63(1): P. 35-42.
9. Csiszar, N. Labinskyy, X. Zhao et al.Vascular superoxide and hydrogen peroxide production and oxidative stress resistance in two closely related rodent species with disparate longevity// Aging Cell 2007. V.6. š 6. P. 783797.
10. Gavrilov L. A. and Gavrilova N. S., The Biology of Life Span: A Quantitative Approach. New York: Harwood Academic Publisher - 1991.
11. Landgraf M, Thor S. Development of Drosophila motoneurons: specification and morphology// Semin Cell Dev Biol. 2006 V.17. N. 1. P. 3-11. Epub 2005 Dec 13.
12. Parkes T.L., Elia A.J., Dickinson D. et al. Extension of Drosophila lifespan by overexpression of human SOD1 in motorneurons. // Nat Genet. 1998 N.2. P. 171-174.
13. Remolina SC, Hughes KA. Evolution and mechanisms of long life and high fertility in queen honey bees//Age (Dordr). 2008 V.30. N.(2-3). P.177-185.
14. Rodier F., Campisi J., Bhaumik D. Two faces of p53: aging and tumor suppression.// Nucleic Acids Res. 2007, V.35. N.22. P. 7475-7484.
15. Szilard L. On the nature of the aging process.// Proc Natl Acad Sci U S A. 1959 V.45. N. P. 30-45.
16. Wicks S., Bain N., Duttaroy A. Hilliker A.J., Phillips J.P. Hypoxia rescues early mortality conferred by superoxide dismutase deficiency.// Free Radic Biol Med. 2009 V.46. N.2. P. 176-181.